Mathematicians! I need help! (Matrices / General solution to simultaneous equations)

engstrom0304 · 21 May 2013 at 20:26

Hey Guys...

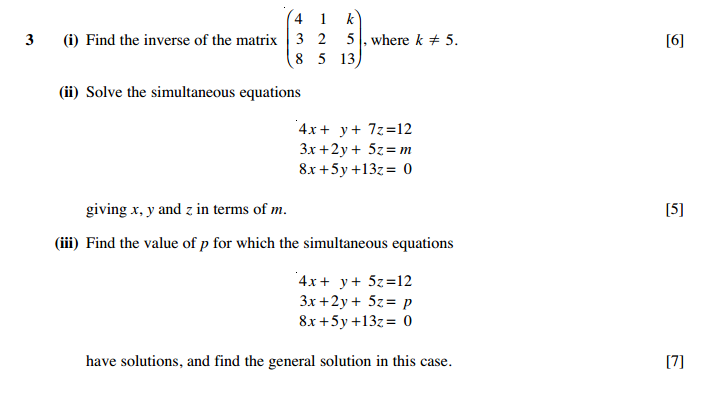
I'm a little stuck on this question and was wondering if you guys could give me a hand.

It is part iii) that I am not sure about. You can see that the simultaneous equation is based on the matrix in part i) but with k = 5 ...

This means that the determinant is 0... so I think i'm right in saying that there are either no solutions or infinitely many solutions.

Part iii has me stuck. I can see how it has been done (from the mark scheme) but am struggling to see why that works. How do you know if there are no solutions or infinitely many if you have 3 simultaneous equations where determinant is 0?

Mark Scheme:

Amleto · 21 May 2013 at 23:51

if you have zero determinant, then you just plug a constant 'k', into what you have left. You either get a contradiction => no solution, or you get an answer for your variables in terms of k => infinite solutions.

Cliste · 22 May 2013 at 00:01

muon · 22 May 2013 at 00:03

Philtor · 22 May 2013 at 00:36

Not sure but pie = 3.14 if that helps?

pingwing · 22 May 2013 at 00:37

Burn it all and cry witchcraft

Iraklis F.C. · 22 May 2013 at 00:50

if i remember well, if det A=0 then det A= -det A ?

gregorius · 22 May 2013 at 08:15

Think geometrically about what a matrix equation is. A 3x3 matrix corresponds to a linear transformation mapping T: R^3 --> R^3. If the determinant of the matrix is non-zero then the transformation has an inverse, and the solution of the matrix equation is obtained by multiplying the rhs by the inverse of the matrix.

If the determinant of the matrix is zero then the linear transformation is not invertible. Since it's a linear transformation this means that T:R^3-->S, where S is some strict linear subset of R3 (a plane, a line or a point). The matrix equation will only have a solution if the vector on the right hand side lies in S. If the rhs does lie in S, then the inverse image of the rhs will be a line or a plane (or all of R^3 in the degenerate case) in R^3.

Illgresi · 22 May 2013 at 08:26

Might have sums wrong, but i got determinant as 64..

Illgresi · 22 May 2013 at 08:30

det A = 4((2*13)-(5*5))-((3*13)-(5*8))+((3*5)-(2*8))

gregorius · 22 May 2013 at 08:40

Illgresi said:
det A = 4((2*13)-(5*5))-((3*13)-(5*8))+5((3*5)-(2*8))

Corrected for you...

Illgresi · 22 May 2013 at 08:43

Ah, been up since 3 :/

booyaka · 22 May 2013 at 09:45

pingwing said:
Burn it all and cry witchcraft

that make me chuckle. thanks!

Iraklis F.C. · 22 May 2013 at 12:47

Illgresi said:
det A = 4((2*13)-(5*5))-((3*13)-(5*8))+((3*5)-(2*8))

but all that is equal to zero? i dont know it got me confused because he says detA=0 , that means its not solvable in my books.

gregorius · 22 May 2013 at 13:57

Iraklis F.C. said:
but all that is equal to zero? i dont know it got me confused because he says detA=0 , that means its not solvable in my books.

Det(A)=0 means that it will only be solvable if the vector on the rhs of the equation is in the image of A.